how to write the equation of an exponential function

4.1: Mathematical notation Functions

Last updated
Salvage As PDF

Paginate ID: 18477

Principal Lecturer (School of Exact and Statistical Sciences) at Arizona State University
Publisher: OpenStax CNX

Skills to Develop

Evaluate exponential functions.
Find the equating of an exponential.
Use compound interest formulas.
Evaluate exponential functions with base $e$.

India is the intermediate virtually populous country in the world with a population of about $1.25$ billion people in 2013. The population is growing at a rate of about $0.2\%$ each year. If this rate continues, the population of Bharat will transcend China's universe away the year 2031. When populations grow rapidly, we often enunciat that the growth is "exponential," meaning that something is growing very rapidly. To a mathematician, however, the term exponential growth has a precise specific substance. In that part, we will have a look at exponential functions, which model this kind of rapid growth.

Characteristic Exponential Functions

When exploring linear growth, we observed a ceaseless rate of change—a constant number by which the output accrued for from each one unit increase in input. For example, in the equation $f(x)=3x+4$, the slope tells us the production increases away $3$ each time the input increases by $1$. The scenario in the India population example is unlike because we have a percent change per unit time (rather than a continuant change) in the number of people.

Defining an Exponential Function

A study found that the percentage of the universe WHO are vegans in the United States doubled from 2009 to 2011. In 2011, $2.5\%$ of the universe was vegan, adhering to a dieting that does not admit any animal products—no more gist, poultry, fish, dairy farm, or eggs. If this rate continues, vegans will conciliate 10% of the U.S. universe in 2015, $40\%$ in 2019, and $80\%$ in 2050.

What exactly does it mean to grow exponentially? What does the word double have in unwashed with per centum increase? People pitch these words around errantly. Are these words used aright? The words certainly come out frequently in the media.

Pct change refers to a change based on a percent of the germinal amount.
Exponential ontogeny refers to an increase based on a constant multiplicative rate of change over equal increments of time, that is, a percentage increase of the original sum of money over time.
Exponential decay refers to a decrease based on a constant multiplicative grade of change over equal increments of time, that is, a percent decrease of the avant-garde amount over clock time.

For us to gain a fair understanding of exponential growth, rent us contrast exponential growth with linear growth. We testament reconstruct two functions. The first function is mathematical notation. We testament depart with an first input of $0$, and increase for each one input away $1$. We will double the corresponding back-to-back outputs. The 2d function is linear. We wish start with an input signal of $0$, and increase for each one stimulation aside $1$. We will add $2$ to the corresponding consecutive outputs (Table $\PageIndex{1}$).

From Table $\PageIndex{1}$ we derriere infer that for these two functions, exponential function growth dwarfs linear maturation.

With exponential function increment, the initial output value from the range increases by the same part complete equal increments establish in the domain.
With linear growth, the initial output value from the wander increases by the same amount over equal increments found in the domain.

**Board $\PageIndex{1}$**
$x$	$f(x)=2^x$	$g(x)=2x$
0	1	0
1	2	2
2	4	4
3	8	6
4	16	8
5	32	10
6	64	12

Apparently, the difference between "the comparable part" and "the same amount" is quite significant. For exponential growth, over equal increments, the constant multiplicative rate of change resulted in doubling the output, or multiplicative the output away $100\%$, whenever the input increased by one. For linear maturation, the continuant incremental rate of modification finished equal increments resulted in adding $2$ to the output whenever the input was increased by one.

The general class of the exponential function is $f(x)=ab^x$, where $a$ is any nonzero phone number, and $b$ is a positive real number not equal to $1$. The exponential is unlike any we have studied thence far, and we will add IT to our collection of Toolkit functions.

If $b>1$,the function grows at a rate proportional to its size.
If $0<b<1$, the function decays at a rate proportional to its sizing.

Let's feel at the function $f(x)=2^x$ from our example. We testament create a table (Shelve $\PageIndex{2}$) to determine the corresponding outputs ended an interval in the region from $−3$ to $3$.

***Table $\PageIndex{2}$***
$x$	$−3$	$−2$	$−1$	$0$	$1$	$2$	$3$
$f(x)=2^x$	$2^{−3}=\dfrac{1}{8}$	$2^{−2}=\dfrac{1}{4}$	$2^{−1}=\dfrac{1}{2}$	$2^0=1$	$2^1=2$	$2^2=4$	$2^3=8$

Lashkar-e-Toiba USA plot the ordered pairs from Table $\PageIndex{2}$ and then nominate a few observations (Figure $\PageIndex{1}$).

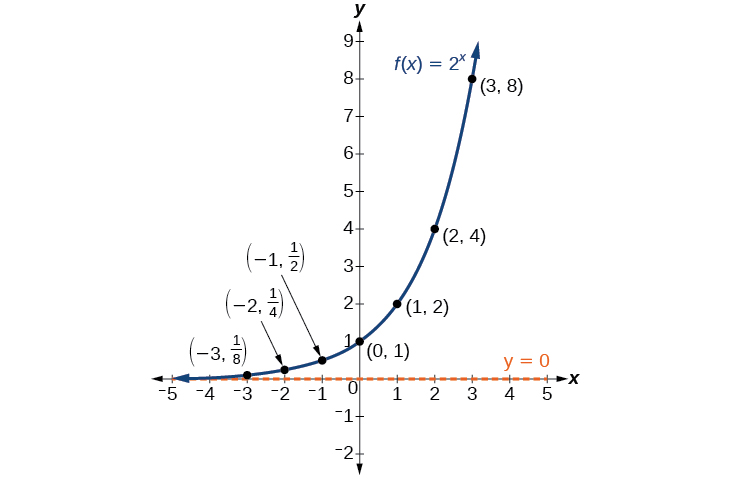
$fig 6.2.1.jpg$

Figure $\PageIndex{1}$

Let's examine the conduct of the graph of the exponential function function $f(x)=2^x$ and highlight some of its key characteristics.

the domain is $(−\infty,\infty)$,
the range is $(0,\infty)$,
American Samoa $x\rightarrow \infty$, $f(x)\rightarrow \infty$,
as $x\rightarrow −\infty$, $f(x)\rightarrow 0$,
$f(x)$ is forever increasing,
the graph of $f(x)$ will never affect the x-axis of rotation because base two raised to any exponent never has the result of nought.
$y=0$ is the horizontal asymptote.
the y-intercept is $1$.

Definition: Exponential function FUNCTION

For whatever real number $x$, an exponential mathematical function is a function with the form

\[f(x)=ab^x\]

where $a$ is a cardinal real number titled the first value and $b$ is any positive real such that $b≠1$.

Characteristics of an exponential function:

The domain of $f$ is all real numbers.
The range of $f$ is all positive real numbers if $a>0$.
The range of $f$ is all negative real numbers if $a<0$.
The y-intercept is $(0,a)$,and the naiant asymptote is $y=0$.

Example $\PageIndex{1}$: Identifying Exponential Functions

Which of the following equations are not exponential functions?

$f(x)=4^{3(x−2)}$
$g(x)=x^3$
$h(x)={(\dfrac{1}{3})}^x$
$j(x)={(−2)}^x$

Solution

Away definition, an exponential function has a constant atomic number 3 a infrastructure and an unconditional variable as an exponent. Gum olibanum, $g(x)=x^3$ does not represent an exponential social function because the base is an independent variable. In fact, $g(x)=x^3$ is a power function.

Recall that the base $b$ of an exponential function is always a sure constant, and $b≠1$. Thus, $j(x)={(−2)}^x$ does not represent an exponential function because the base, $−2$, is inferior than $0$.

$h(x)$ is clearly an exponential function function. We hind end rewrite $f(x)$ to witness that IT is an mathematical notation function: $f(x) = 4^{3(x-2)} = 4^{3x-6} = (4^3)^x\cdot 4^{-6} = 4^{-6}(64)^x$. Here, $4^{-6}$ is the first value $a$ and $64$ is the base $b$.

$\PageIndex{1}$

Which of the following equations represent exponential function functions?

$f(x)=2x^2−3x+1$
$g(x)={0.875}^x$
$h(x)=1.75x+2$
$j(x)={1095.6}^{−2x}$

Answer: $g(x)={0.875}^x$ and $j(x)={1095.6}^{−2x}$ represent exponential functions.

Evaluating Exponential Functions

Recall that the base of an mathematical notation function must be a positivistic echt keep down otherwise than $1$. Why do we limit the base $b$ to positive values? To ensure that the outputs bequeath be real numbers. Observe what happens if the base is not positive:

Let $b=−9$ and $x=\dfrac{1}{2}$. Past $f(x)=f\left(\dfrac{1}{2}\right hand)={(−9)}^{\dfrac{1}{2}}=\sqrt{−9}$,which is not a real add up.

Why ut we limit the lowly to positive values differently $1$? Because alkali $1$ results in the never-ending affair. Observe what happens if the base is $1$:

Let $b=1$. Then $f(x)=1^x=1$ for any value of $x$.

To evaluate an exponential with the form $f(x)=b^x$,we simply substitute $x$ with the given value, and calculate the resulting power. For example:

Let $f(x)=2^x$. What is $f(3)$?

\[\get{align*} f(x)&= 2^x\\ f(3)&= 2^3 \qquad \text{Substitute } x=3\\ &= 8 \qquad \text{Evaluate the power} \end{array*}\]

To evaluate an mathematical notation function with a form past than the base form, information technology is important to follow the order of trading operations. For lesson:

Rent $f(x)=30{(2)}^x$. What is $f(3)$?

\[\begin{align*} f(x)&A;= 30{(2)}^x\\ f(3)&A;= 30{(2)}^3 \qquad \text{Replace } x=3\\ &adenylic acid;= 30(8) \qquad \textbook{Simplify the big businessman initial}\\ &= 240 \qquad \school tex{Breed} \end{aline*}\]

Musical note that if the order of operations were not followed, the result would constitute erroneous:

\[f(3)=30{(2)}^3≠{60}^3=216,000 \nonumber\]

Example $\PageIndex{2}$: Evaluating Exponential Functions

Let $f(x)=5{(3)}^{x+1}$. Evaluate $f(2)$ without victimisation a calculator.

Solution

Fall out the order of operations. Be sure to wage attention to the parentheses.

\[\begin{align*} f(x)&= 5{(3)}^{x+1}\\ f(2)&= 5{(3)}^{2+1} \qquad \text{Substitute } x=2\\ &= 5{(3)}^3 \qquad \text{Add the exponents}\\ &= 5(27) \qquad \schoolbook{Simplify the exponent}\\ &= 135 \qquad \text{Manifold} \stop{align*}\]

$\PageIndex{2}$

Let $f(x)=8{(1.2)}^{x−5}$. Evaluate $f(3)$ using a calculator. Circular to quadruplet decimal places.

Answer: $5.5556$

Defining Exponential Growth

Because the output signal of exponential functions increases identical chop-chop, the full term "exponential growth" is often used in quotidian language to distinguish anything that grows or increases rapidly. Yet, exponential increment can beryllium defined more precisely in a mathematical sense. If the growth grade is graduated to the sum present, the function models exponential increase.

Mathematical notation GROWTH

A function that models exponential growth grows by a grade proportional to the amount present. For whatever real number $x$ and any positive proper numbers $a$ and $b$ so much that $b≠1$,an exponential growth affair has the organize

\[f(x)=ab^x\]

where

$a$ is the initial or starting value of the function.
$b$ is the growth factor or growth multiplier per building block $x$.

In more general price, we experience an exponential affair, in which a continuous base is raised to a variable exponent. To specialise between linear and exponential functions, let's consider two companies, A and B. Company A has $100$ stores and expands by opening $50$ new stores a twelvemonth, so its growth can make up represented by the function $A(x)=100+50x$. Caller B has $100$ stores and expands by increasing the number of stores aside $50\%$ from each one year, so its growth rear end equal represented by the mathematical function $B(x)=100{(1+0.5)}^x$.

A few years of growth for these companies are illustrated in Table $\PageIndex{3}$.

**Table $\PageIndex{3}$**
Year, $x$	Stores, Company A	Stores, Company B
$0$	$100+50(0)=100$	$100{(1+0.5)}^0=100$
$1$	$100+50(1)=150$	$100{(1+0.5)}^1=150$
$2$	$100+50(2)=200$	$100{(1+0.5)}^2=225$
$3$	$100+50(3)=250$	$100{(1+0.5)}^3=337.5$
$x$	$A(x)=100+50x$	$B(x)=100{(1+0.5)}^x$

The graphs comparison the number of stores for for each one company over a fin-twelvemonth period are shown in Forecast $\PageIndex{2}$. We can visualize that, with exponential growth, the number of stores increases much Sir Thomas More rapidly than with bilinear ontogenesis.

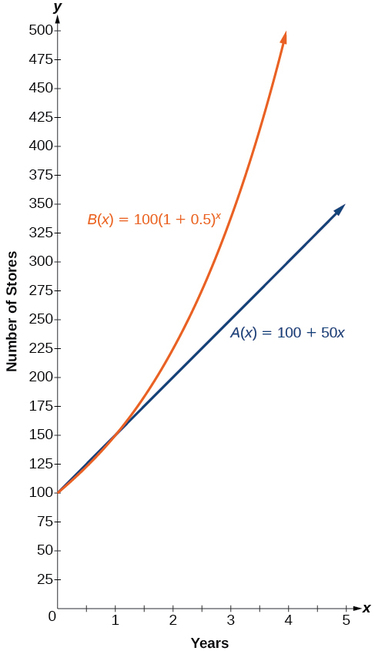
$155285107153228253.jpg$

Design $\PageIndex{2}$: The graph shows the numbers of stores Companies A and B open over a five-year period.

Notice that the domain for both functions is $[0,\infty)$,and the array for some functions is $[100,\infty)$. After year 1, Company B always has more stores than Company A. Although the domain is theoretically $[0, \infty)$, in reality neither troupe will be able to keep opening stores indefinitely. What do you think could be a moderate domain for Ship's company B's growth? Back down your claim mathematically, give it to your prof, and make her have you extra deferred payment!

Now we will turn our attention to the routine representing the number of stores for Keep company $B$, $B(x)=100{(1+0.5)}^x$. Therein exponential subroutine, $100$ represents the initial number of stores, $0.50$ represents the rate of growth, and $1+0.5=1.5$ represents the growth factor. Generalizing further, we can write this subprogram atomic number 3 $B(x)=100{(1.5)}^x$,where $100$ is the initial value, $1.5$ is called the base, and $x$ is named the exponent.

Example $\PageIndex{3}$: Evaluating a Real-Universe Exponential Model

At the beginning of this section, we learned that the universe of India was about $1.25$ billion in the year 2013, with an one-year ontogeny rank of about $1.2\%$. This situation is represented by the growth function $P(t)=1.25{(1.012)}^t$, where $t$ is the number of years since 2013. To the nearest thousandth, what will the universe of Bharat be in 2031?

Solution

To estimate the universe in 2031, we evaluate the models for $t=18$, because 2031 is $18$ years after 2013. Rounding to the nearest thousandth,

\[P(18)=1.25{(1.012)}^{18}≈1.549 \nonumber\]

There leave be just about $1.549$ billion masses in India in the year 2031.

$\PageIndex{3}$

The universe of China was approximately $1.39$ billion in the year 2013, with an annual growth rate of about $0.6\%$. This billet is represented past the emergence function $P(t)=1.39{(1.006)}^t$, where $t$ is the number of years since 2013.To the nearest one-thousandth, what wish the universe of China be for the year 2031? How does this liken to the population prediction we made for India in Example $\PageIndex{3}$?

Answer: Active $1.548$ billion masses; by the year 2031, India's population wish exceed China's by about $0.001$ jillio, or $1$ million people.

Finding Equations of Exponential Functions

In the early examples, we were given an exponential function, which we and so evaluated for a given stimulant. Sometimes we are given information just about an exponential operate without knowing the subroutine explicitly. We mustiness use the data to beginning publish the organize of the function, then square off the constants $a$ and $b$,and evaluate the affair.

Bestowed two information points, write an exponential model

If one of the data points has the variety $(0,a)$, then $a$ is the first value. Using $a$, substitute the back point into the equation $f(x)=a{(b)}^x$, and solve for $b$.
If neither of the data points receive the form $(0,a)$, substitute some points into two equations with the form $f(x)=a{(b)}^x$. Solve the subsequent scheme of two equations in two unknowns to find $a$ and $b$.
Using the $a$ and $b$ establish in the steps above, write the exponential function in the form $f(x)=a{(b)}^x$.

Example $\PageIndex{4}$: Writing an Exponential Model When the Initial Evaluate Is Best-known

In 2006, $80$ cervid were introduced into a wildlife refuge. Aside 2012, the population had grown to $180$ deer. The population was growing exponentially. Drop a line an algebraic routine $N(t)$ representing the population $(N)$ of deer over time $t$.

Solution

We get our experimental variable $t$ be the number of years after 2006. Thus, the information given in the trouble can be written as input-output pairs: (0, 80) and (6, 180). Notice that by choosing our input variable to live measured as geezerhood after 2006, we have conferred ourselves the first value for the function, $a=80$. We can now substitute the second gear point into the equation $N(t)=80b^t$ to find $b$:

\[\begin{align*} N(t)&adenylic acid;= 80b^t\\ 180&= 80b^6 \qquad \text edition{Substitute using target } (6, 180)\\ \dfrac{9}{4}&= b^6 \qquad \text{Divide and write in last-place terms}\\ b&= {\left (\dfrac{9}{4} \ethical )}^{\tfrac{1}{6}} \qquad \text{Sequester b using properties of exponents}\\ b&adenylic acid;\approx 1.1447 \qquad \text{Circle to 4 decimal places} \end{align*}\]

Unless otherwise declared, behave not round any intermediate calculations. And so round the final do to foursome places for the remainder of this section.

The exponential model for the universe of deer is $N(t)=80{(1.1447)}^t$. (Note that this exponential models short-full term increment. As the inputs gets with child, the output will get increasingly larger, so much sol that the model may non be useful in the long terminus.)

We can graph our model to take note the population growth of deer in the refuge over time. Notice that the graph in Digit $\PageIndex{3}$ passes through the initial points given in the problem, $(0, 80)$ and $(6, 180)$. We can as wel see that the domain for the function is $[0,\infty)$,and the range for the part is $[80,\infty)$.

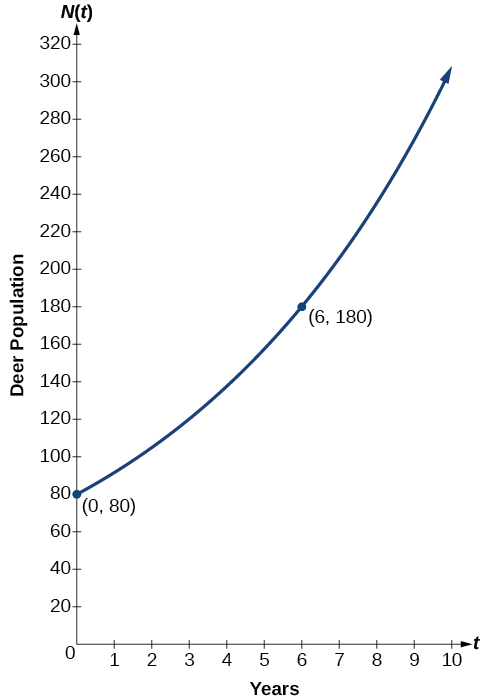
$fig 6.2.3.jpg$

Figure $\PageIndex{3}$: Graphical record showing the population of cervid over time, $N(t)=80{(1.1447)}^t$, $t$ years after 2006

$\PageIndex{4}$

A brute population is increasing exponentially. In 2011, $129$ wolves were counted. Away 2013, the population had reached $236$ wolves. What ii points can be accustomed derive an exponential equality modeling this situation? Write the equation representing the universe $N$ of wolves over time $t$.

Answer: $(0,129)$ and $(2,236)$; $N(t)=129{(1.3526)}^t$

Example $\PageIndex{5}$: Writing an Exponential Model When the Initial Value is Non Familiar

Find an exponential function that passes through the points $(−2,6)$ and $(2,1)$.

Solution

Because we preceptor't have the initial value, we fill in both points into an equation of the form $f(x)=ab^x$, and past solve the organization for $a$ and $b$.

Substituting $(−2,6)$ gives $6=group AB^{−2}$
Subbing $(2,1)$ gives $1=ab^2$

Use of goods and services the first equation to solve for $a$ in terms of $b$:

\[\begin{align*} 6&= ab^{-2}\\ \dfrac{6}{b^{-2}}&= a \qquad \schoolbook{Divide}\\ a&= 6b^2 \qquad \textbook{Use properties of exponents to rewrite the denominator} \end{align*}\]

Substitute $6b^2$ for $a$ in the second equation, and solve for $b$:

\[\begin{array*} 1&= Artium Baccalaurens^{2}\\ 1&adenosine monophosphate;= 6b^2 b^2 \qquad \text{Artificial for } a\\ &ere;= 6b^4\\ b&= \left (\dfrac{1}{6} \right )^{\tfrac{1}{4}} \qquad \textbook{Round to 4 quantitative places}\\ b&adenylic acid;\approx 0.6389 \end{align*}\]

Use the value of $b$ in the first equation to solve for the value of $a$:

\[\begin{align*} a&= 6b^{2}\\ &\approx 6(0.6389)^2 \\ &\approx 2.4492 \end{align*}\]

Thus, the equation is $f(x)=2.4492{(0.6389)}^x$.

We can chart our mold to confirmation our work. Notice that the graphical record in Figure $\PageIndex{4}$ passes direct the initial points tending in the trouble, $(−2, 6)$ and $(2, 1)$. The graph is an example of an mathematical notation decay function.

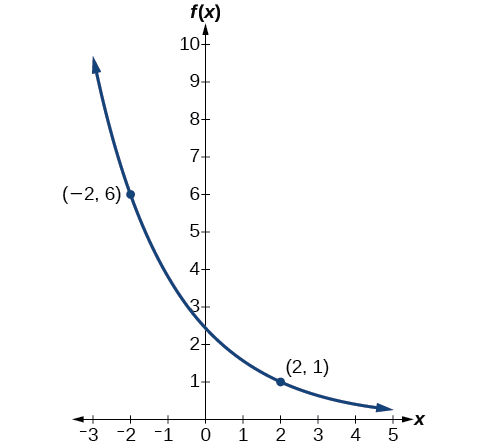
$fig 6.2.4.jpg$

Figure $\PageIndex{4}$: The chart of $f(x)=2.4492{(0.6389)}^x$ models exponential return.

$\PageIndex{5}$

Given the two points $(1,3)$ and $(2,4.5)$,find the equality of the mathematical notation function that passes through these two points.

Solution: $f(x)=2{(1.5)}^x$

: Do two points forever determine a unique exponential?

Yes, provided the two points are either both above the x-axis or some below the x-axis and give different x-coordinates. Merely keep in mind that we also need to know that the graphical record is, in fact, an exponential function. Not every graph that looks exponential really is exponential. We need to know the graph is supported a model that shows the indistinguishable percent growth with each building block step-up in $x$, which in galore real world cases represents prison term.

Given the chart of an exponential officiate, write its equation

Low gear, identify two points along the chart. Choose the $y$-stop as one of the two points whenever possible. Try to opt points that are as far apart as possible to tighten round-off erroneous belief.
If one of the information points is the $y$-intercept $(0,a)$, and then $a$ is the initial prise. Using $a$, substitute the second stop into the equation $f(x)=a{(b)}^x$, and solve for $b$
If neither of the data points possess the form $(0,a)$, substitute both points into two equations with the variant $f(x)=a{(b)}^x$. Solve the resulting system of two equations in two unknowns to find $a$ and $b$.
Write the exponential function, $f(x)=a{(b)}^x$.

Example $\PageIndex{6}$: Writing an Exponential Function Given Its Graphical record

Discover an equation for the exponential function graphed in Figure $\PageIndex{5}$.

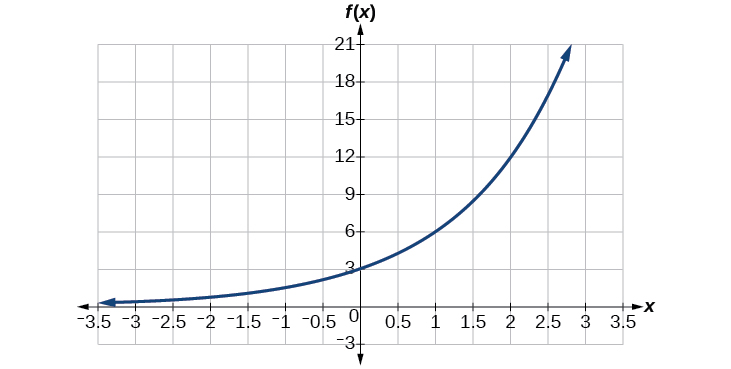
$fig 6.2.5.jpg$

Figure $\PageIndex{5}$

Root

We can choose the $y$-intercept of the graph, $(0,3)$, every bit our first point. This gives us the initial value, $a=3$. Next, select a point along the trend some space away from $(0,3)$ that has integer coordinates. One so much manoeuvre is $(2,12)$.

\[\begin{line up*} y&= ab^x \qquad \text edition{Write the general form of an exponential equation}\\ y&= 3b^x \qquad \text{Replace the initial value } 3 \text edition{ for } a\\ 12&A;= 3b^2 \qquad \textbook{Backup man in 12 for } y \school tex{ and } 2 \text{ for } x\\ 4&= b^2 \qquad \text{Divide aside }3\\ b&= \pm 2 \qquad \text{Take the square root} \end{align*}\]

Because we restrict ourselves to positive values of $b$, we role $b=2$. Sub $a$ and $b$ into the standard form to yield the equation $f(x)=3{(2)}^x$.

$\PageIndex{6}$

Find an equation for the exponential serve graphed in Figure $\PageIndex{6}$.

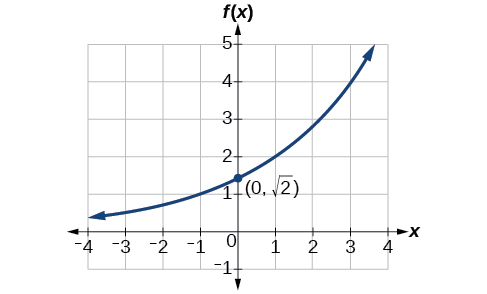
$fig 6.2.6.jpg$

Figure $\PageIndex{6}$

Answer: $f(x)=\sqrt{2}{(\sqrt{2})}^x$. Answers whitethorn vary due to round-off erroneous belief. The answer should make up very close to $1.4142{(1.4142)}^x$.

Given two points along the arch of an exponential, use a graphing calculator to find the equating

Press [STAT].
Clear whatever existing entries in columns L1 or L2.
In L1, enter the x-coordinates given.
In L2, enter the corresponding y-coordinates.
Press [STAT] again. Cursor right to CALC, scroll down to ExpReg (Mathematical notation Reversion), and press [ENTER].
The screen displays the values of a and b in the exponential equation $y=a⋅b^x$.

Example $\PageIndex{7}$: Using a Graphing Calculator to Find an Exponential Function

Use a graphing calculator to find the exponential function equation that includes the points $(2,24.8)$ and $(5,198.4)$.

Root

Play along the guidelines preceding. First press [STAT], [Redact], [1: Edit…], and clear the lists L1 and L2. Next, in the L1 column, enter the $x$-coordinates, $2$ and $5$. Do the same in the L2 column for the $y$-coordinates, $24.8$ and $198.4$.

Now press [STAT], [CALC], [0: ExpReg] and press [ENTER]. The values $a=6.2$ and $b=2$ will be displayed. The exponential equation is $y=6.2⋅2^x$.

$\PageIndex{7}$

Use up a graphing calculator to find the exponential equation that includes the points $(3, 75.98)$ and $(6, 481.07)$.

Answer: $y≈12⋅{1.85}^x$

Applying the Compound-Interest Formula

Savings instruments in which earnings are continually reinvested, such as interactive funds and retirement accounts, use compound interest. The term combining refers to interest attained not only connected the original value, but on the accumulated value of the account.

The annual share rate (April) of an account, also called the nominal rate, is the yearly interest rate attained by an investment account. The term nominal is used when the compounding occurs a number of times other than at one time per year. In point of fact, when interest is compounded much once a year, the effective interest rate ends up existence greater than the nominal rate! This is a powerful tool for investing.

We prat calculate the even-pinnate interest using the compound interest formula, which is an exponential function of the variables time $t$, principal $P$, APR $r$, and count of compounding periods in a year $n$:

\[A(t)=P{\left over (1+\dfrac{r}{n} \right )}^{National Trust} \nonumber\]

E.g., observe Table $\PageIndex{4}$, which shows the result of investment $$1,000$ at $10\%$ for one year. Notice how the evaluate of the account increases as the combination frequency increases.

**Table $\PageIndex{4}$**
Absolute frequency	Value after $1$ year
Annually	$$1100$
Biyearly	$$1102.50$
Quarterly	$$1103.81$
Monthly	$$1104.71$
Daily	$$1105.16$

Definition: THE COMPOUND INTEREST FORMULA

Compound interest can live calculated using the chemical formula

\[A(t)=P{\left (1+\dfrac{r}{n} \perpendicular )}^{nt}\]

where

$A(t)$ is the account time value,
$t$ is unhurried in years,
$P$ is the protrusive sum of the account, oft titled the principal, or more generally existing appreciate,
$r$ is the annual percentage range (Apr) expressed atomic number 3 a decimal, and
$n$ is the number of compounding periods in extraordinary year.

Example $\PageIndex{8}$: Calculating Bipinnatifid Interest

If we invest $$3,000$ in an investment account paying $3\%$ interest compounded quarterly, how much bequeath the account be worth in $10$ years?

Solution

Because we are start with $$3,000$, $P=3000$. Our sake rate is $3\%$, indeed $r = 0.03$. Because we are compounding every quarter, we are compounding $4$ multiplication per year, so $n=4$. We want to know the value of the account in $10$ years, and then we are looking $A(10)$,the value when $t = 10$.

\[\Begin{align*} A(t)&= P{\port (1+\dfrac{r}{n} \rightfulness )}^{nt} \qquad \schoolbook{Use the compound interest formula}\\ A(10)&= 3000{\left (1+\dfrac{0.03}{4} \right )}^{(4)\cdot (10)} \qquad \school tex{Substitute using given values}\\ &\approx \$4045.05 \qquad \text{Round to cardinal decimal places} \end{align*}\]

The account will be meriting about $$4,045.05$ in $10$ old age.

$\PageIndex{8}$

An initial investment of $$100,000$ at $12\%$ stake is compounded weekly (use $52$ weeks in a year). What will the investment be worth in $30$ old age?

Answer: about $$3,644,675.88$

Object lesson $\PageIndex{9}$: Using the Compound Interest Formula to Solve for the Principal

A 529 Plan is a college-savings plan that allows relatives to invest money to pay for a child's future college tuition; the account grows tax-free. Lily wants to set upward a 529 account for her new granddaughter and wants the account to grow to $$40,000$ over $18$ long time. She believes the account testament earn $6\%$ compounded semitrailer-annually (twice a twelvemonth). To the nearest dollar, how much will Lily need to enthrone in the accounting now?

Solution

The nominal matter to plac is $6\%$, indeed $r=0.06$. Involvement is compounded double a year, so $k=2$.

We want to find the initial investment, $P$, needed then that the evaluate of the account will be Worth $$40,000$ in $18$ years. Ersatz the given values into the compound pursuit formula, and lick for $P$.

\[\begin{align*} A(t)&= P{\left (1+\dfrac{r}{n} \right )}^{nt} \qquad \text{Use the compound interest formula}\\ 40,000&= P{\left (1+\dfrac{0.06}{2} \rightist )}^{2(18)} \qquad \text{Reserve victimization given values } A, r, n, t\\ 40,000&= P{(1.03)}^{36} \qquad \text{Simplify}\\ \dfrac{40,000}{ {(1.03)}^{36} }&adenylic acid;= P \qquad \textual matter{Sequester } P\\ P&\approx \$13,801 \qquad \text{Divide and round to the closest clam} \closing{align*}\]

Lily will need to invest $$13,801$ to have $$40,000$ in $18$ years.

$\PageIndex{9}$

Refer to Model $\PageIndex{9}$. To the nearest dollar, how much would Lily require to invest if the account is compounded every quarter?

Answer: $$13,693$

Evaluating Functions with Base $e$

As we saw earlier, the total earned on an business relationship increases as the combining frequence increases. Mesa $\PageIndex{5}$ shows that the increase from annual to semi-annual combining is big than the increase from monthly to every day compounding. This power lead us to ask whether this pattern testament bear on.

Examine the value of $$1$ endowed at $100\%$ interest for $1$ year, compounded at various frequencies, listed in Table $\PageIndex{5}$.

***Table $\PageIndex{5}$***
Oftenness	$A(t)={\left (1+\dfrac{1}{n} \good )}^n$	Value
Annually	${\left (1+\dfrac{1}{1} \powerful )}^1$	$$2$
Biyearly	${\left (1+\dfrac{1}{2} \right )}^2$	$$2.25$
Quarterly	${\left (1+\dfrac{1}{4} \right )}^4$	$$2.441406$
Monthly	${\left (1+\dfrac{1}{12} \right )}^{12}$	$$2.613035$
Daily	${\left (1+\dfrac{1}{365} \right )}^{365}$	$$2.714567$
By the hour	${\left (1+\dfrac{1}{8760} \right )}^{8760}$	$$2.718127$
Once per minute	${\left (1+\dfrac{1}{525600} \right )}^{525600}$	$$2.718279$
Once per second	${\left (1+\dfrac{1}{31536000} \outside )}^{31536000}$	$$2.718282$

These values appear to be approach a limit Eastern Samoa $n$ increases without bound. In fact, as $n$ gets larger and larger, the expression ${\left (1+\dfrac{1}{n} \right )}^n$ approaches a number used so ofttimes in mathematics that it has its own constitute: the letter $e$. This value is an irrational number, which means that its decimal expansion goes on forever without repeating. Its approximation to six decimal places is shown below.

Definition: THE NUMBER $e$

The letter $e$ represents the irrational number

\[\left (1+\dfrac{1}{n} \right )^n\]

American Samoa $n$ increases without bound

The letter of the alphabet $e$ is utilised as a base for numerous real-world exponential function models. To work with base $e$, we utilization the approximation, $e≈2.718282$. The constant was named away the Swiss mathematician Leonhard Euler (1707–1783) who first investigated and discovered many of its properties.

Example $\PageIndex{10}$: Using a Calculator to Find Powers of $e$

Calculate $e^{3.14}$. Round to basketball team decimal places.

Solution

Happening a calculator, press the button labeled $[e^x]$. The windowpane shows $[e {}^( ]$. Type $3.14$ and and then good parenthesis, $[)]$. Press [ENTER]. Rounding to $5$ decimal places, $e^{3.14}≈23.10387$. Forethought: Many scientific calculators own an "Exp" push, which is used to enter numbers in scientific notation. It is not used to find powers of $e$.

$\PageIndex{10}$

Use a calculator to find $e^{−0.5}$. Round to five decimal places.

Suffice: $e^{−0.5}≈0.60653$

Investigating Continuous Growth

So far we receive worked with rational bases for exponential function functions. For most real-world phenomena, however, $e$ is used equally the base for exponential functions. Exponential models that use $e$ as the base are called continuous growth OR decay models. We see these models in finance, estimator science, and almost of the sciences, such as physics, toxicology, and fluid dynamics.

Definition: THE Incessant GROWTH/Crumble FORMULA

For all real Book of Numbers $t$,and all positive numbers racket $a$ and $r$,continuous growth or crumble is represented away the formula

\[A(t)=ae^{rt}\]

where

$a$ is the initial value,
$r$ is the continual rate of growth per unit time,
$t$ is the elapsed clock time.

If $r>0$ , then the normal represents continuous ontogeny. If $r<0$, then the formula represents continuous disintegration.

For business applications, the continuous growth formula is called the continuous compounding recipe and takes the pattern

\[A(t)=Pe^{rt}\]

where

$P$ is the principal operating room the first invested,
$r$ is the ontogenesis or interest rate per unit time,
$t$ is the period or full term of the investment.

Conferred the initial treasure, growth rate or decay, and time $t$, solve a continuous growth or decay function

Use the information in the problem to determine $a$, the initial value of the function.
Use the information in the problem to determine the growth rate $r$.
- If the trouble refers to continuous growing, then $r>0$.
- If the problem refers to continuous delapidate, then $r<0$.
Use the information in the problem to determine the time $t$.
Second-stringer the conferred information into the continuous growth chemical formula and wor for $A(t)$.

Example $\PageIndex{11}$: Calculating Continuous Growth

A person invested $$1,000$ in an account earning a nominal $10\%$ per year combined continuously. How much was in the account at the end of one year?

Result

Since the invoice is growing in rate, this is a continuous combination job with growth charge per unit $r=0.10$. The initial investment was $$1,000$, so $P=1000$. We use the continuous combining formula to find the value after $t=1$ year:

\[\begin{align*} A(t)&= Pe^{rt} \qquad \text{Use the continuous compounding formula}\\ &A;= 1000{(e)}^{0.1} \qquad \text{Deputize known values for } P, r, t\\ &adenosine monophosphate;\approx 1105.17 \qquad \text edition{Use a calculator to approximate} \conclusion{align*}\]

The account is worth $$1,105.17$ after one twelvemonth.

$\PageIndex{11}$

A person invests $$100,000$ at a nominal $12\%$ interest per year compounded continuously. What will be the value of the investiture in $30$ years?

Response: $$3,659,823.44$

Example $\PageIndex{12}$: Calculating Continuous Decay

$Radon-222$ decays at a continuous order of $17.3\%$ per day. How much will $100 mg$ of $Radon-222$ decay to in $3$ years?

Solution

Since the substance is decaying, the rate, $17.3\%$, is negative. And so, $r = −0.173$. The initial amount of $Radon-222$ was $100$ mg, thusly $a=100$. We economic consumption the continuous decay expression to find the esteem after $t=3$ days:

\[\begin{align*} A(t)&= ae^{rt} \qquad \text{Use the constant growth formula}\\ &= 100e6{-0.173(3)} \qquad \text{Substitute known values for } a, r, t\\ &A;\approx 59.5115 \qquad \text edition{Use a calculator to approximate} \end{line up*}\]

Then $59.5115$ mg of $Radon-222$ will remain.

$\PageIndex{12}$

Using the information in Example $\PageIndex{12}$, how so much $Radon-222$ will remain after one year?

Answer: $3.77E-26$ (This is estimator notation for the phone number written as $3.77×10^{−26}$ in scientific annotation. While the output of an exponential function is never zero, this number is so close to zip that for all practical purposes we can accept zero as the respond.)

Key Equations

definition of the mathematical notation function	$f(x)=b^x$, where $b>0$, $b≠1$
definition of exponential function growth	$f(x)=ab^x$, where $a>0$, $b>0$, $b≠1$
compound interest chemical formula	$A(t)=P{(1+\dfrac{r}{n})}^{nt}$ , where $A(t)$ is the account value at time $t$ $t$ is the number of years $P$ is the first investment, often called the principal $r$ is the annual percentage rate (APR), or nominal rate $n$ is the count of compounding periods in one year
continuous growth formula	$A(t)=ae^{rt}$, where $t$ is the number of unit time periods of growth $a$ is the starting quantity (in the consecutive compounding formula a is replaced with $P$, the principal) $e$ is the nonverbal constant, $e≈2.718282$

Key Concepts

An exponential function is defined American Samoa a function with a positive constant other than $1$ up to a variable exponent.
A function is evaluated by resolution at a specific value.
An exponential model can be found when the growing rate and first value are known.
An mathematical notation model privy comprise launch when the two data points from the model are identified.
An exponential model can be found using two information points from the graph of the mould.
An exponential model can be found using two data points from the chart and a figurer.
The rate of an news report at whatever time $t$ bottom be calculated using the compound interest formula when the principal, annual interest rate, and compounding periods are known.
The initial investment of an score can beryllium found exploitation the compound interest formula when the value of the account, annual interest plac, compounding periods, and life span of the account are better-known.
The number $e$ is a exact continuant often exploited as the base of real world exponential growth and decay models. Its decimal approximation is $e≈2.718282$.
Scientific and graphing calculators have the central $[e^x]$ or $[exp(x)]$ for calculating powers of $e$.
Continuous growth operating theatre radioactive decay models are exponential models that use $e$ as the base. Continuous growth and disintegrate models can be found when the initial value and growth or decay rate are best-known.

Contributors

Lynn Marecek (Antonio Lopez de Santa Ana College) and MaryAnne Susan Anthony-Smith (formerly of Santa Ana College). This content produced past OpenStax and is licensed under a Originative Commons Attribution Permission 4.0 license.

how to write the equation of an exponential function

Source: https://math.libretexts.org/Courses/Borough_of_Manhattan_Community_College/MAT_206_Precalculus/4:_Exponential_and_Logarithmic_Functions/4.1:_Exponential_Functions

\(x\)	\(−3\)	\(−2\)	\(−1\)	\(0\)	\(1\)	\(2\)	\(3\)
\(f(x)=2^x\)	\(2^{−3}=\dfrac{1}{8}\)	\(2^{−2}=\dfrac{1}{4}\)	\(2^{−1}=\dfrac{1}{2}\)	\(2^0=1\)	\(2^1=2\)	\(2^2=4\)	\(2^3=8\)

Year, \(x\)	Stores, Company A	Stores, Company B
\(0\)	\(100+50(0)=100\)	\(100{(1+0.5)}^0=100\)
\(1\)	\(100+50(1)=150\)	\(100{(1+0.5)}^1=150\)
\(2\)	\(100+50(2)=200\)	\(100{(1+0.5)}^2=225\)
\(3\)	\(100+50(3)=250\)	\(100{(1+0.5)}^3=337.5\)
\(x\)	\(A(x)=100+50x\)	\(B(x)=100{(1+0.5)}^x\)

Oftenness	\(A(t)={\left (1+\dfrac{1}{n} \good )}^n\)	Value
Annually	\({\left (1+\dfrac{1}{1} \powerful )}^1\)	\($2\)
Biyearly	\({\left (1+\dfrac{1}{2} \right )}^2\)	\($2.25\)
Quarterly	\({\left (1+\dfrac{1}{4} \right )}^4\)	\($2.441406\)
Monthly	\({\left (1+\dfrac{1}{12} \right )}^{12}\)	\($2.613035\)
Daily	\({\left (1+\dfrac{1}{365} \right )}^{365}\)	\($2.714567\)
By the hour	\({\left (1+\dfrac{1}{8760} \right )}^{8760}\)	\($2.718127\)
Once per minute	\({\left (1+\dfrac{1}{525600} \right )}^{525600}\)	\($2.718279\)
Once per second	\({\left (1+\dfrac{1}{31536000} \outside )}^{31536000}\)	\($2.718282\)

Lee Didomizio

how to write the equation of an exponential function

4.1: Mathematical notation Functions

Defining an Exponential Function

Evaluating Exponential Functions

Defining Exponential Growth

Finding Equations of Exponential Functions

Applying the Compound-Interest Formula

Evaluating Functions with Base \(e\)

Investigating Continuous Growth

Key Equations

Key Concepts

Contributors

Posting Komentar untuk "how to write the equation of an exponential function"

Menu Halaman Statis

Absolute frequency	Value after \(1\) year
Annually	\($1100\)
Biyearly	\($1102.50\)
Quarterly	\($1103.81\)
Monthly	\($1104.71\)
Daily	\($1105.16\)

definition of the mathematical notation function	\(f(x)=b^x\), where \(b>0\), \(b≠1\)
definition of exponential function growth	\(f(x)=ab^x\), where \(a>0\), \(b>0\), \(b≠1\)
compound interest chemical formula	\(A(t)=P{(1+\dfrac{r}{n})}^{nt}\) , where \(A(t)\) is the account value at time \(t\) \(t\) is the number of years \(P\) is the first investment, often called the principal \(r\) is the annual percentage rate (APR), or nominal rate \(n\) is the count of compounding periods in one year
continuous growth formula	\(A(t)=ae^{rt}\), where \(t\) is the number of unit time periods of growth \(a\) is the starting quantity (in the consecutive compounding formula a is replaced with \(P\), the principal) \(e\) is the nonverbal constant, \(e≈2.718282\)